Fractions are one of the most important mathematical concepts that we teach our students. It’s also a topic that students often struggle with, and one that teachers tend to dread teaching. In this blog, we’ve compiled seven tips to help make teaching fractions a more effective and enjoyable experience for you and your students. But first, let’s look at why it’s such a crucial concept to master.

Why Is Teaching Fractions So Important?

While foundational fractional concepts and operations are taught at the elementary level, fractions are a critical component of advanced math studies. For example, an understanding of fractions is necessary when determining the slope of a line, evaluating rational expressions, calculating statistical probabilities, and verifying trigonometric identities. Aside from math classes, students will likely work with fractions in other subject areas, such as dimensional analysis in chemistry or physics.

Having a strong foundation in fractions isn’t just important for higher education, though. It’s important for life in general. Students will encounter fractions frequently in their daily lives, like when following a recipe, measuring something, or figuring out a discounted price.

7 Tips for Teaching Fractions Successfully

Teaching fractions is far more effective and enjoyable once you realize why your students are struggling and how they learn best. Here are seven tips to help your students master this important math subject once and for all.

1) Help Students See Fractions as Numbers

It can be challenging for students to understand fractions at first because their math knowledge so far is built on whole numbers. Introducing fractions flips their world upside down a bit, and now they must grapple with the fact that an infinite number of numbers exist between any two whole numbers.

Additionally, because students are used to thinking in terms of whole numbers, they may not comprehend that the numerator and denominator represent one value rather than two unique numbers. Be sure to model correct mathematical vocabulary to your students while teaching. When working with 2/3, say “two-thirds.” Saying “the top number” and “the bottom number” can perpetuate the misconception that the numerator and denominator have two different values rather than one collective value.

2) Use Visual Models

Fractions may seem abstract to students without visuals to help them see the relationship between the parts and the whole. To encourage a deep, foundational knowledge of fractions, you must first provide concrete and representational models. This gives your students a visual that they can internalize and call upon to create their own mental models in the future.

We recommend using a variety of visuals when teaching fractions, even within the same activity. Some commonly-used fraction models include:

• Area models such as tape diagrams and circle diagrams
• Measurement models such as number lines and fraction strips 
• Set models where you divide a set of objects into equal subsets

Our Math-U-See manipulatives, specifically the Fraction Overlay Kit, are valuable tools to help your students visualize fractions and build conceptual understanding with models.

3) Explain the Importance of Equal-Sized Parts

Students who struggle with fractions sometimes don’t realize that a fraction is part of a whole and that visually the parts have to be equal in size. This is why common denominators are necessary when adding fractions. For example, we add 1/4 + 2/4 rather than 1/4 + 1/2. Referring to the model below, a student may incorrectly label the fraction of shaded parts as 2/3 instead of 2/4 (or 1/2) if they aren’t reminded to divide the figure into parts of equal size.

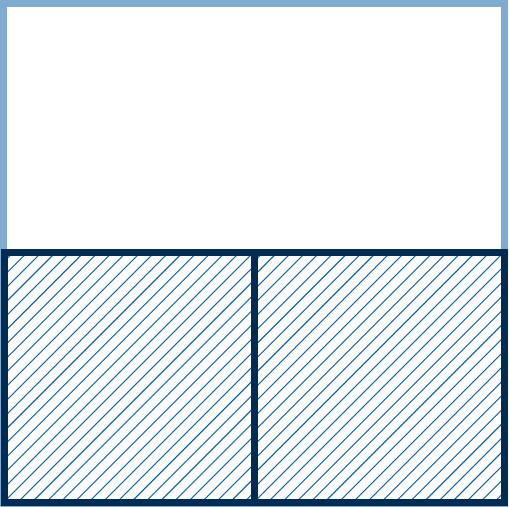
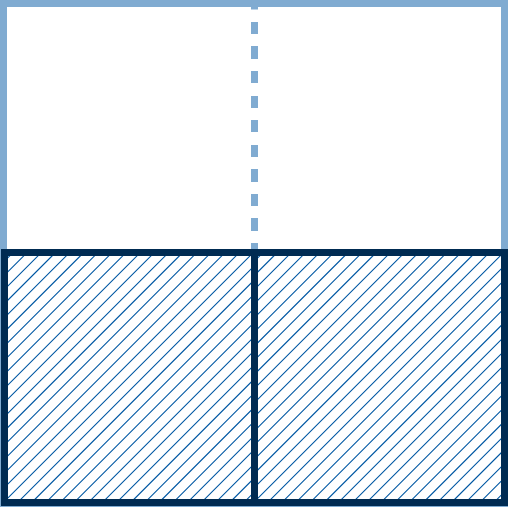
The dashed line shown in the figure above needs to be added to the figure by your student so that the fraction is divided into equal parts.

4) Show the Size of Fractions

Because students are used to thinking in whole-number terms, it’s understandable that they often have a hard time comprehending the size of a fraction. For example, since 8 is greater than 4, many students think that 1/8 is greater than 1/4 when it’s actually much smaller when comparing parts of the same whole.

However, simply telling students that having a larger denominator means that a fraction is smaller will create more problems down the road, especially when they must compare fractions such as 8/9 and 2/3. This is another situation where using models and making real-world connections helps, like showing the difference between 1/8 and 1/4 of a pizza.

5) Encourage Estimation

Estimation helps students get a good grasp on how big or small a fraction is. The ability to estimate also helps students be more successful when comparing fractions and performing operations with fractions. 

Using number lines teaches students to form a visual in their head, which they can use to make an estimation. Be sure to teach them how to use reference points like 1/4, 1/2, and 3/4 to guide their understanding while using a number line.

6) Foster Strong Fraction Sense First

When teaching fractions, don’t rush right into the rules and algorithms. Instead, take time to develop fraction sense—a deep understanding of fractions—before jumping into procedures involving fractions.

In order to develop a strong fraction sense, you must teach your students the meaning behind fraction concepts rather than relying on memorization. Too often, teachers jump right into operational methods, such as cross multiplication, without having students explore how and why it works. This causes students to apply procedures blindly and often leads to confusion.

Using whole number operation rules is a good indicator that your student doesn’t have sufficient fraction sense. Spend more time reviewing foundational skills and providing more visual models before readdressing the rules for working with fractions.

7) Practice, Practice, Practice

Just like any skill, proficiency with fractions requires practice. So, be sure to provide your students with plenty of opportunities to “flex their fraction muscles.” We suggest making real-world connections and incorporating your students’ interests as much as possible. 

For example, if your student likes football, have them determine the fraction of games their favorite team has won this season. This will pique their interest and help them see the relevance of what they’re learning.

Teaching fractions is no simple task, but we hope these seven tips will help make the process much smoother and more successful.

Are you a parent of a student who’s struggling with fractions? Watch this video for some additional insights!